



At his website Atlantis, Dr. Karl Scherer introduced similar or scaled tetrads. These are arrangements of four similar shapes in which each borders the other three.
In general, scaled tetrads are easier to find that standard tetrads. Scherer's page showed how the right tromino can form scaled tetrads. The smallest polyiamond that can form standard tetrads has three cells.
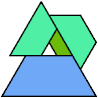
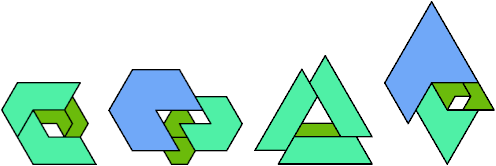
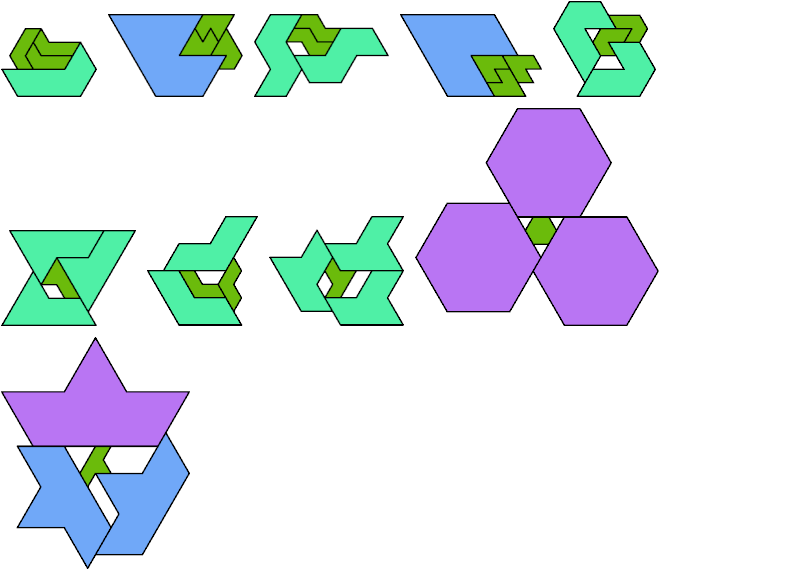
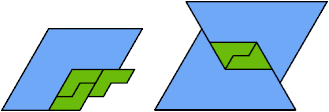
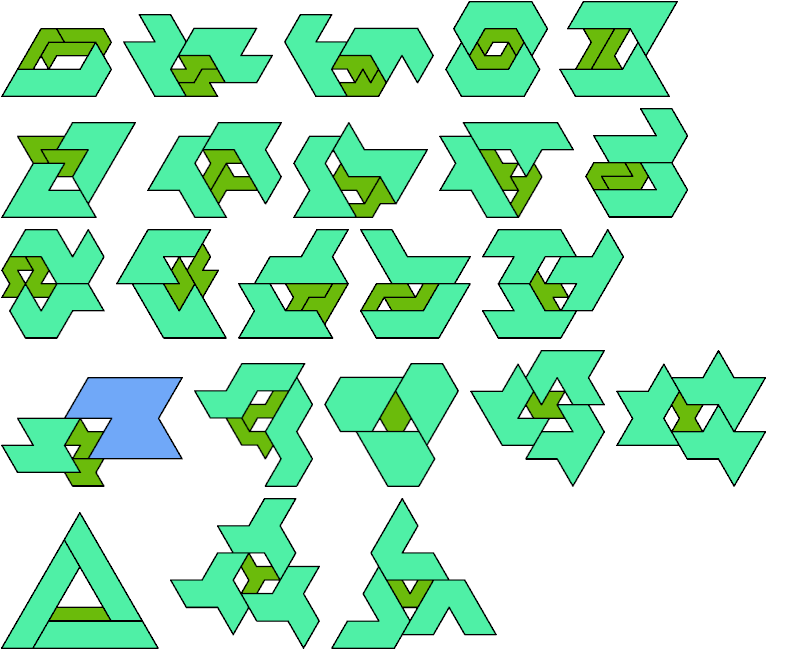
Scherer proved that convex shapes cannot form tetrads. However, many can form scaled tetrads, as shown below.
Here I show the smallest known scaled tetrads for polyiamonds with up to 7 cells. If you find a smaller solution or solve an unsolved case, please write.
See also Scaled Polytan Tetrads.

| 
| 
| 
|
| 1 | 2 | 3 | 4 |
|---|

Last revised 2023-05-18.