Stelo

  • Introduction
  • Triplets
  • Convex Twins

    • Introduction

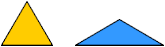
    Stelo is Jacques Ferroul's set of 12 pieces, which he calls the triapons. A triapons is formed by joining three cells on the polydrafter grid. Each cell may be an equilateral triangle, a moniamond, or an isosceles triangle with base angles of 30°, a monopons.

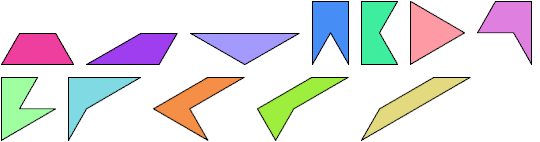
    These are the 12 triapons:

    Polyiapons may be regarded as a special case of proper polydrafters. In particular, the triapons are hexadrafters.

    Stelo is Esperanto for star. Ferroul named the set in memory of his grandniece Maïlou.

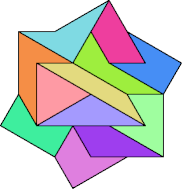
    A Lucite set of Stelo is available from Kadon Enterprises.

    Triplets

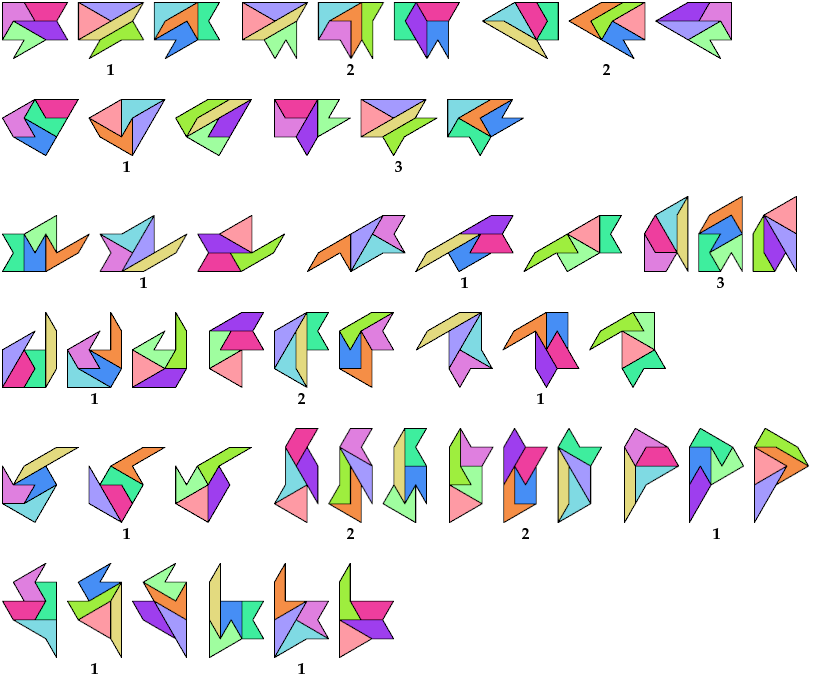
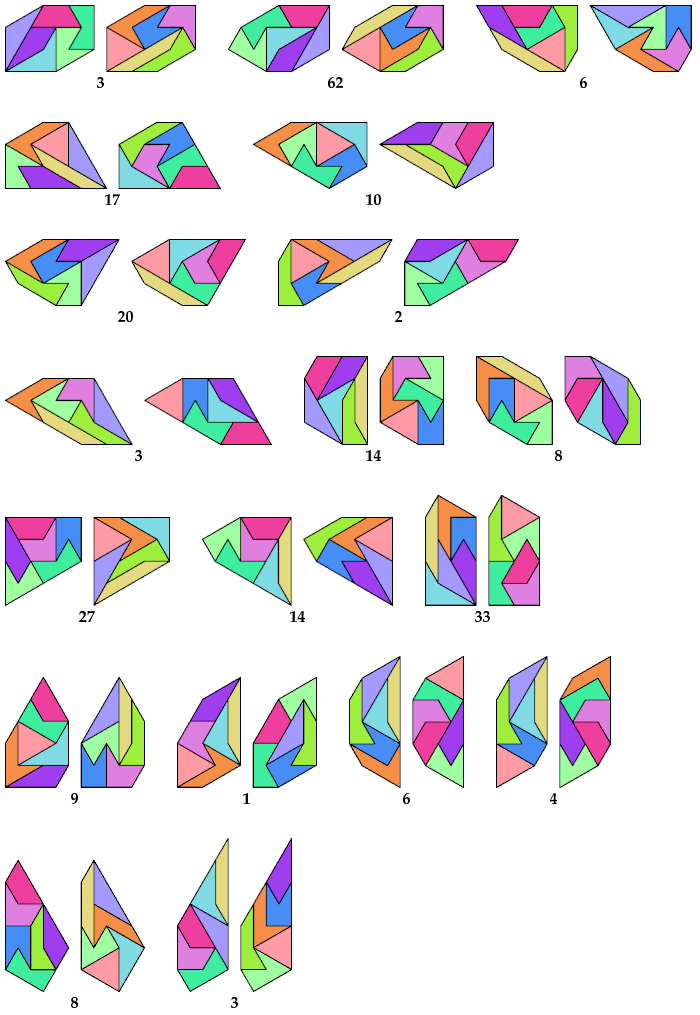
    A set of three identical shapes is called triplets. Stelo can form 17 sets of triplets. The number below each triplet shows the number of distinct tilings.

    Convex Twins

    A set of two identical shapes is called twins. Stelo can form 19 sets of convex twins. The number below each twin shows the number of distinct tilings.

    Last revised 2021-02-08.

    Back to Polydrafter Tiling < Polyform Tiling < Polyform Curiosities
    Col. George Sicherman [ HOME | MAIL ]