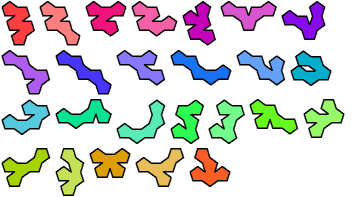
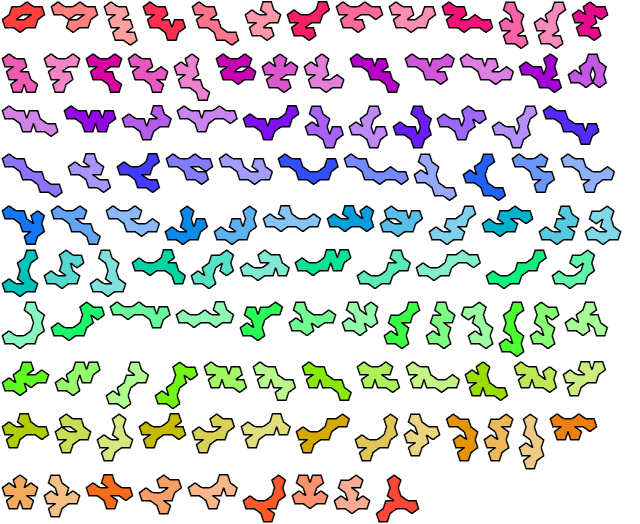
Free polypents are classified by congruence, with no cell used as a base. Two-sided polypents may be rotated and reflected. One-sided polypents may be rotated but not reflected.
| Order | Free Two-Sided | Free One-Sided |
|---|---|---|
| 1 | 1 | 1 |
| 2 | 1 | 1 |
| 3 | 2 | 2 |
| 4 | 7 | 11 |
| 5 | 25 | 43 |
| 6 | 118 | 223 |
| 7 | 551 | 1 072 |
| 8 | 2 812 | 5 564 |
| 9 | 14 445 | 28 747 |
| 10 | 76 092 | 151 897 |
| 11 | 403 976 | 807 245 |
| 12 | 2 167 116 | 4 332 812 |
Matthias Koch and
Sascha Kurz have since enumerated polypents up through order 16.
See Matthias Koch and Sascha Kurz, Enumeration of Generalized
Polyominoes.
The enumeration for free polypents is A103465 in the On-Line Encyclopedia of Integer Sequences.
The figures below show two-sided polypents.




Last revised 2023-06-02.