2 Tiles
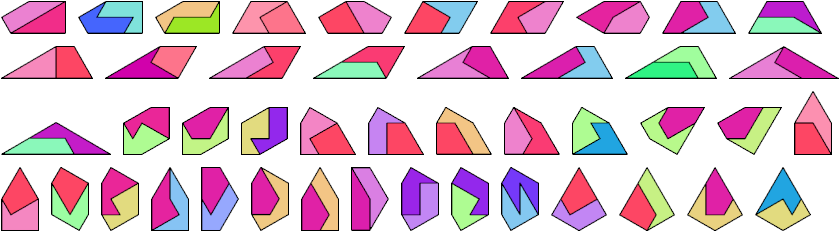
3 Tiles

4 Tiles
5 Tiles

6 Tiles
7 Tiles
8 Tiles

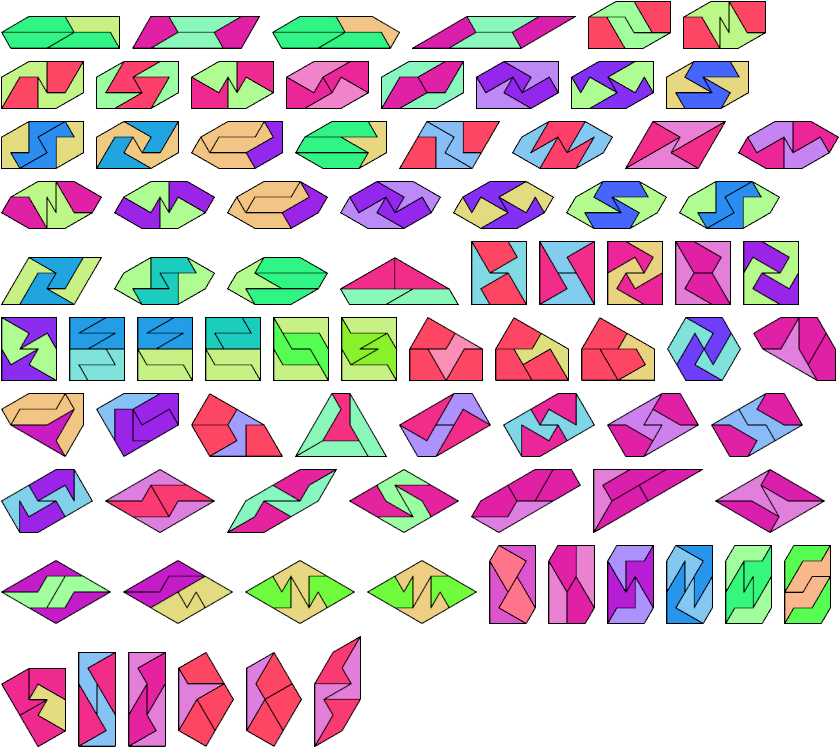
9 Tiles
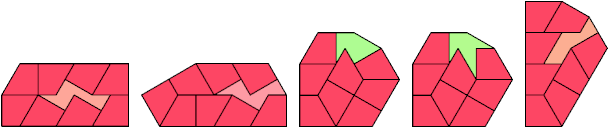
10 Tiles
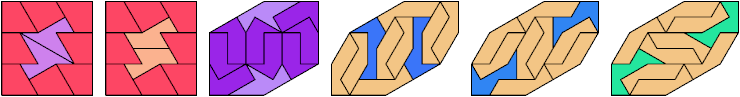
11 Tiles
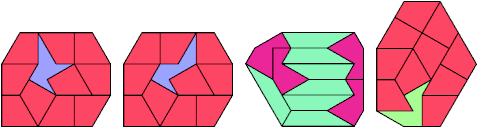
12 Tiles
14 Tiles
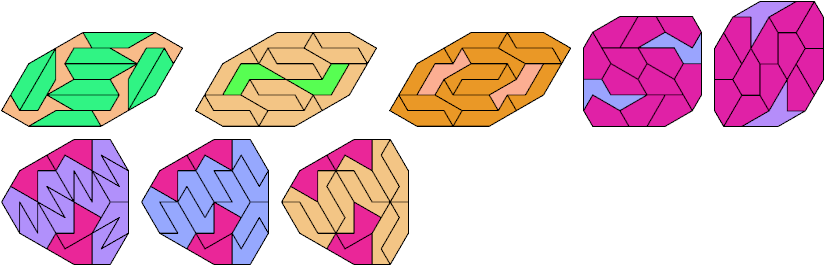
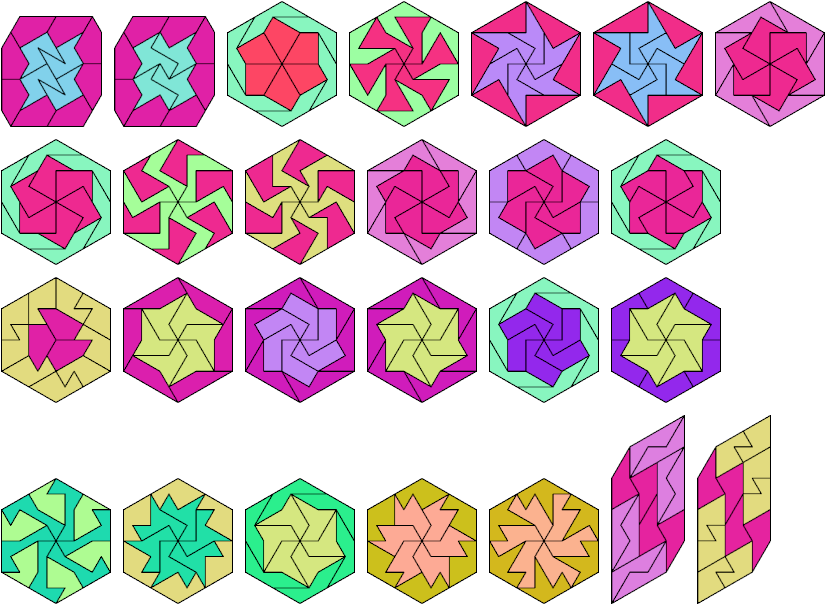
16 Tiles
17 Tiles
18 Tiles
20 Tiles
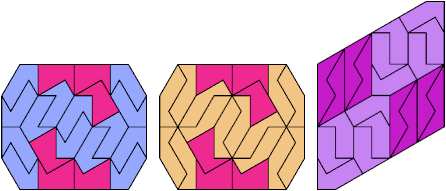
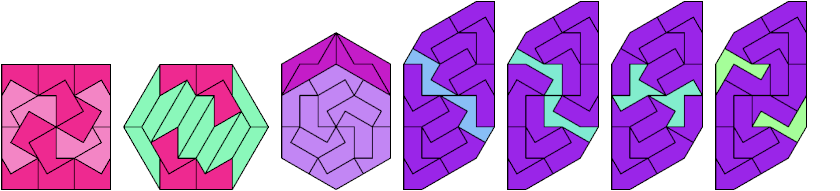
22 Tiles
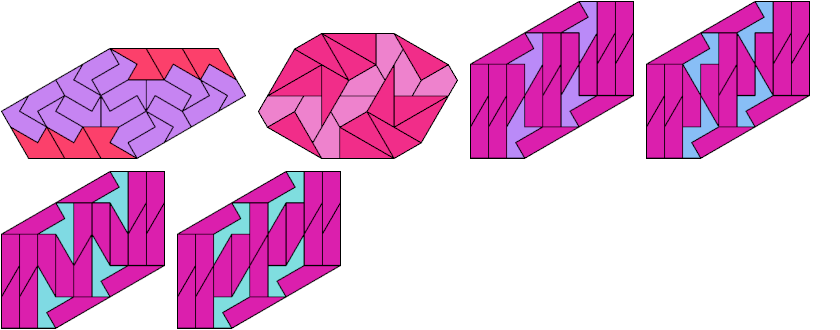
23 Tiles

24 Tiles
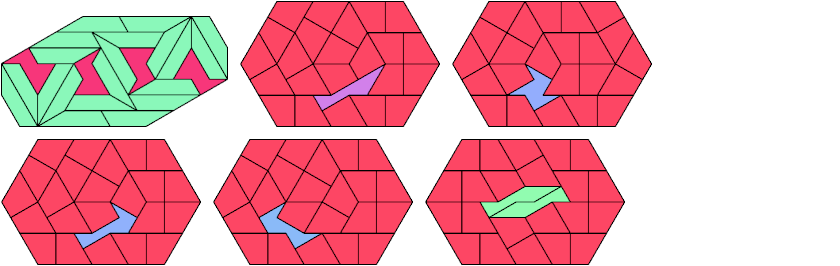
26 Tiles
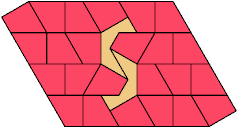
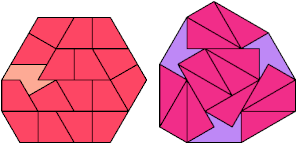
29 Tiles
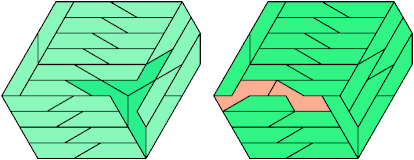
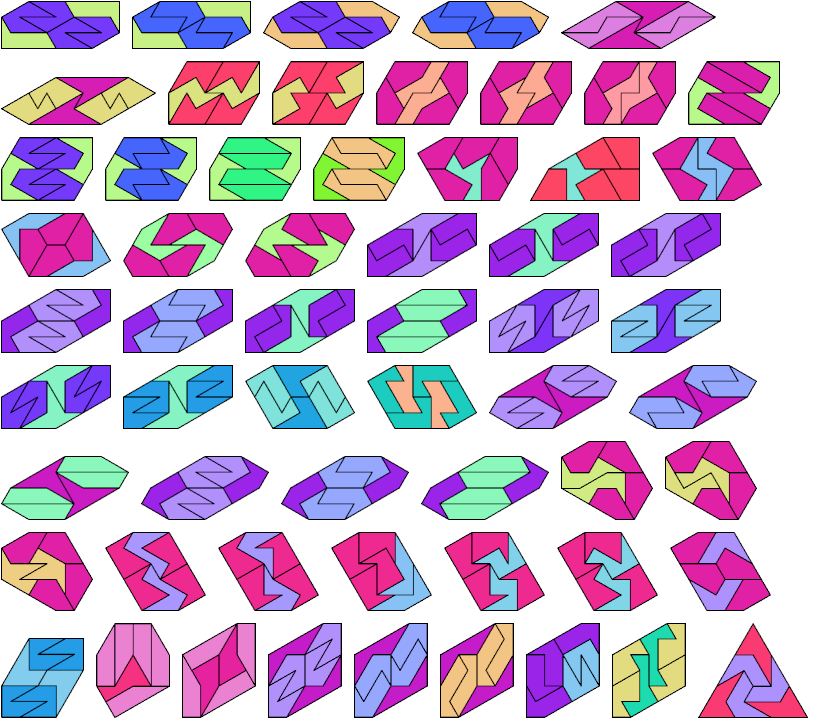
36 Tiles
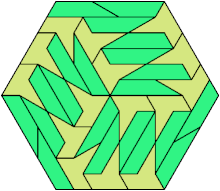
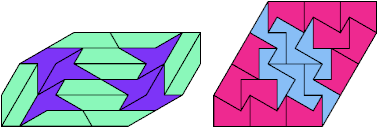
38 Tiles
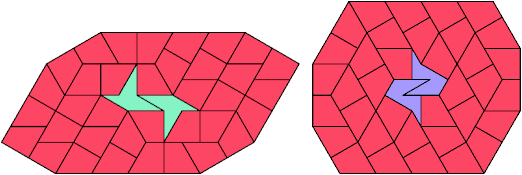
40 Tiles
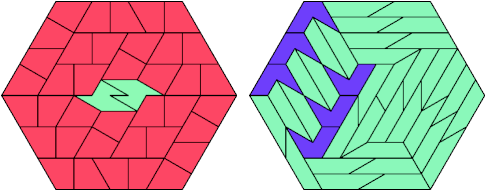
42 Tiles
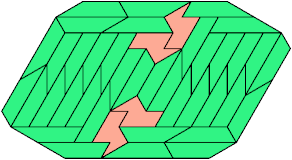
48 Tiles

52 Tiles
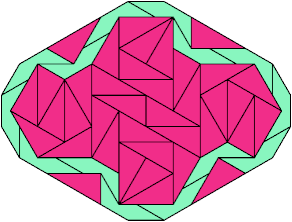
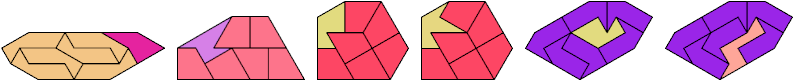
Lost Sheep
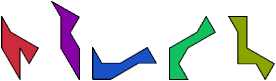
No convex pairing is known for any of these five tridrafters:
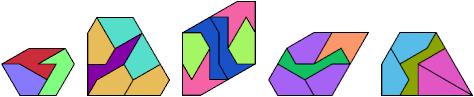
Each can form a convex shape with copies of two other tridrafters:
Last revised 2020-09-08.
Back to Polydrafter Tiling < Polyform Tiling < Polyform Curiosities
Col. George Sicherman [ HOME | MAIL ]