Convex Figures with Didrafter Triplets

Introduction

A didrafter is a polyform made by joining two drafters, 30°-60°-90° right triangles, at their short legs, long legs, hypotenuses, or half hypotenuses. Polydrafters joined on the polyiamond (triangle) grid are called proper polydrafters. Polydrafters whose cells depart from the grid are called extended polydrafters. Here are the 13 didrafters, proper and extended:

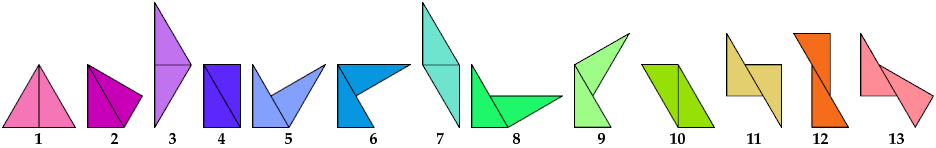
Below I show how to make a minimal convex figure using copies of three didrafters, at least one of each. These solutions are not necessarily unique, nor are their tilings. If you find a solution with fewer tiles, or solve an unsolved case, please write.

See also Convex Figures with Didrafter Pairs.

1-2-35 1-4-105 1-8-103 2-4-74 2-7-127 3-5-66 3-9-104 4-7-918 5-6-138 6-7-1214 7-10-11×
1-2-43 1-4-11× 1-8-11× 2-4-83 2-7-136 3-5-718 3-9-11× 4-7-103 5-7-88 6-7-1316 7-10-12×
1-2-53 1-4-12× 1-8-1211 2-4-93 2-8-95 3-5-86 3-9-12× 4-7-11× 5-7-99 6-8-918 7-10-13×
1-2-63 1-4-13× 1-8-1351 2-4-104 2-8-103 3-5-96 3-9-13× 4-7-12× 5-7-10× 6-8-103 7-11-12×
1-2-75 1-5-65 1-9-103 2-4-117 2-8-119 3-5-106 3-10-11× 4-7-13× 5-7-11× 6-8-1112 7-11-13×
1-2-85 1-5-78 1-9-11× 2-4-125 2-8-127 3-5-11× 3-10-12× 4-8-918 5-7-12× 6-8-1266 7-12-13×
1-2-94 1-5-86 1-9-12× 2-4-134 2-8-135 3-5-12× 3-10-13× 4-8-103 5-7-1390 6-8-138 8-9-103
1-2-105 1-5-95 1-9-13× 2-5-63 2-9-103 3-5-1321 3-11-12× 4-8-1113 5-8-96 6-9-103 8-9-11×
1-2-119 1-5-10× 1-10-11× 2-5-75 2-9-115 3-6-79 3-11-13× 4-8-12× 5-8-103 6-9-118 8-9-12×
1-2-128 1-5-11× 1-10-12× 2-5-83 2-9-1211 3-6-813 3-12-13× 4-8-13× 5-8-11× 6-9-12× 8-9-13×
1-2-139 1-5-129 1-10-13× 2-5-93 2-9-136 3-6-94 4-5-63 4-9-108 5-8-1215 6-9-1312 8-10-115
1-3-44 1-5-1322 1-11-12× 2-5-104 2-10-114 3-6-106 4-5-74 4-9-11× 5-8-1324 6-10-115 8-10-125
1-3-516 1-6-76 1-11-13× 2-5-114 2-10-127 3-6-117 4-5-83 4-9-12× 5-9-103 6-10-125 8-10-135
1-3-69 1-6-818 1-12-13× 2-5-125 2-10-135 3-6-127 4-5-93 4-9-13× 5-9-11× 6-10-135 8-11-12×
1-3-74 1-6-95 2-3-45 2-5-134 2-11-12× 3-6-1372 4-5-105 4-10-115 5-9-1220 6-11-12× 8-11-13×
1-3-87 1-6-107 2-3-54 2-6-75 2-11-1310 3-7-810 4-5-115 4-10-12× 5-9-1314 6-11-13× 8-12-13×
1-3-9× 1-6-116 2-3-65 2-6-85 2-12-137 3-7-919 4-5-1210 4-10-137 5-10-11× 6-12-13× 9-10-118
1-3-103 1-6-129 2-3-77 2-6-96 3-4-55 3-7-104 4-5-138 4-11-12× 5-10-125 7-8-9× 9-10-12×
1-3-11× 1-6-1336 2-3-84 2-6-105 3-4-65 3-7-11× 4-6-74 4-11-13× 5-10-138 7-8-104 9-10-138
1-3-12× 1-7-811 2-3-93 2-6-114 3-4-74 3-7-12× 4-6-83 4-12-13× 5-11-12× 7-8-11× 9-11-12×
1-3-13× 1-7-914 2-3-104 2-6-1211 3-4-84 3-7-13× 4-6-93 5-6-77 5-11-13× 7-8-12× 9-11-13×
1-4-53 1-7-106 2-3-114 2-6-135 3-4-94 3-8-920 4-6-105 5-6-85 5-12-1378 7-8-13× 9-12-13×
1-4-63 1-7-11× 2-3-127 2-7-84 3-4-105 3-8-104 4-6-117 5-6-95 6-7-820 7-9-104 10-11-12×
1-4-75 1-7-12× 2-3-135 2-7-94 3-4-1114 3-8-11× 4-6-125 5-6-106 6-7-910 7-9-11× 10-11-13×
1-4-814 1-7-13× 2-4-53 2-7-103 3-4-1214 3-8-129 4-6-135 5-6-118 6-7-1010 7-9-12× 10-12-13×
1-4-9× 1-8-920 2-4-63 2-7-116 3-4-1314 3-8-1320 4-7-89 5-6-1210 6-7-119 7-9-13× 11-12-13×

3 Tiles

4 Tiles

5 Tiles

6 Tiles

7 Tiles

8 Tiles

9 Tiles

10 Tiles

11 Tiles

12 Tiles

13 Tiles

14 Tiles

15 Tiles

16 Tiles

18 Tiles

19 Tiles

20 Tiles

21 Tiles

22 Tiles

24 Tiles

36 Tiles

51 Tiles

66 Tiles

72 Tiles

78 Tiles

90 Tiles

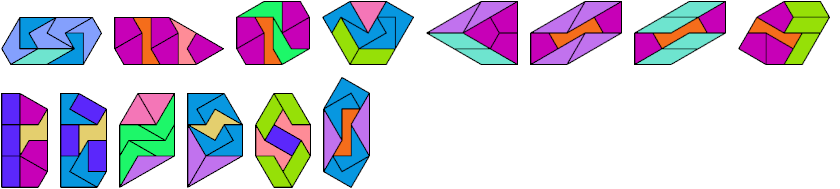
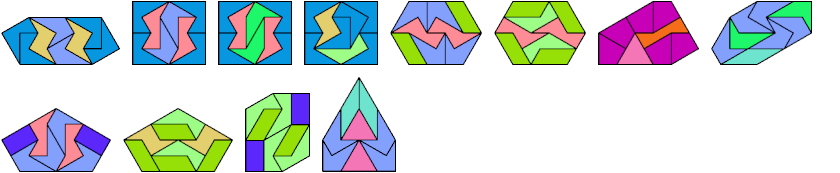
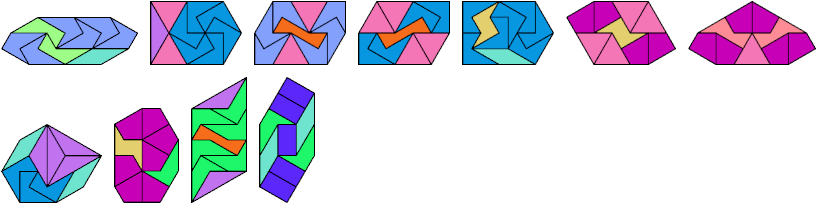
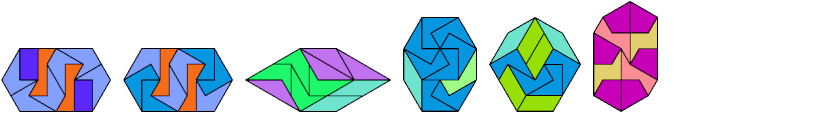
Last revised 2021-04-16.

Back to Polydrafter Tiling < Polyform Tiling < Polyform Curiosities
Col. George Sicherman [ HOME | MAIL ]