Catalogue of
Polypents on the Surface of a Dodecahedron
A polypent
is a figure made by joining regular pentagons edge to edge.
Here I show the different polypents formed by the faces of a
regular dodecahedron.
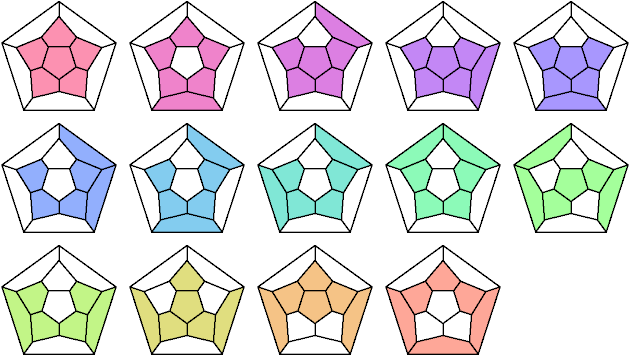
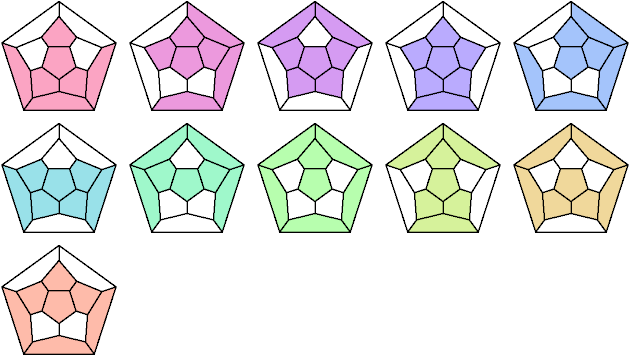
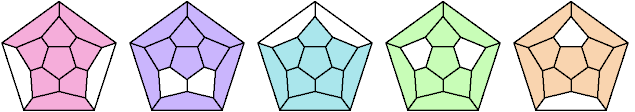
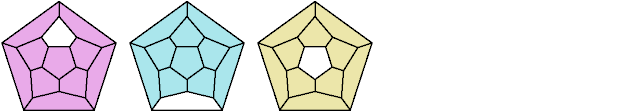
With two-sided polypents, mirror images are identified.
With one-sided polypents, mirror images are distinguished.
In the diagrams below, I show only two-sided polypents.
Thanks to Mark Smith for suggesting this problem.
Enumeration
| Cells | Two-Sided | One-Sided |
|---|
| 1 | 1 | 1 |
| 2 | 1 | 1 |
| 3 | 2 | 2 |
| 4 | 4 | 5 |
| 5 | 7 | 9 |
| 6 | 14 | 20 |
| 7 | 11 | 13 |
| 8 | 10 | 12 |
| 9 | 5 | 5 |
| 10 | 3 | 3 |
| 11 | 1 | 1 |
| 12 | 1 | 1 |
Monopent
Dipent
Tripents
Tetrapents
Pentapents
Hexapents
Heptapents
Octapents
Enneapents
Decapents
Hendecapent
Last revised 2018-03-04.
Back to Polyform Catalogues
<
Polyform Curiosities
Col. George Sicherman
[ HOME
| MAIL
]